Table Of Content
Definition
Centripetal force is an external force required to make a body move along a circular path with uniform speed.
Introduction
When a body moves in a circle, its direction of motion at any instant is represented by a tangent at that instant. It is found that the direction of the moving body in a circle keeps changing continuously. But the magnitude of the velocity remains constant. In this way, the body is said to be performing a Uniform Circular Motion. From Newton’s first law of motion, a body cannot change its direction of motion by itself. Therefore an external force is required to change the direction of motion of the body. This external force is known as ‘Centripetal force’.
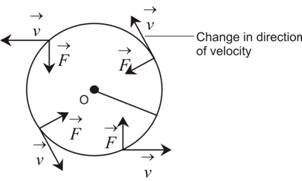
A Particle Moving in a circle
In the above figure, we see a particle moving with a uniform speed ‘v’ in a circular path of radius ‘r’. It has a centripetal acceleration (due to change in direction of velocity) whose magnitude remains constant but direction changes continuously and remains always towards the centre of the circle. Hence it is clear that a body performing circular motion is acted upon by a force which is always directed towards the centre of the circle.This force is called Centripetal Force.
Centripetal Acceleration
When a particle performs a uniform circular motion, its direction changes continuosly though its speed remains constant.Thus,its velocity changes continuosly.That is,circular motion is an accelerated motion.The direction of acceleration is always towards the centre of the circle.Hence it is called Centripetal acceleration.
Suppose a particle is moving with a uniform speed ‘v’ on a circular path of radius ‘r’ with centre ‘O’.

At any instant, the direction of motion of a particle is given by tangent at that instant. Suppose, in a small interval of time ∆t,the particle covers a distance ∆s from P1 to P2 . Let (V1) and (V2) be the velocities of the particle at P1 and P2 respectively. As particle is moving with uniform speed,the magnitude of (V1) and (V2) is V but there is a difference of angle ∆θ in their directions. The change in velocity from P1 to P2 is (V2) – (V1) = ∆(V).
If (V1) and (V2) are drawn from the same point Q (fig. b) and third vector is drawn to complete the vector diagram, this third vector will represent the velocity change (V2) – (V1 ) = ∆(V). The triangle OP1 P2 and the vector triangle QAB are similar.
Hence,
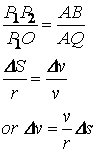
Dividing both sides by ∆t, we get
![]()
If ∆t is infinitesimally small (∆t → 0) then,
![]()

We know that
![]()
therefore
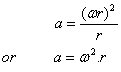
Centripetal Force
When a rigid body moves along a circular path with uniform speed, its direction changes continuously. Due to inertia, at every point of the circular path, the body tends to move along a tangent to the circular path at that point. According to Newton’s Second Law of Motion, a change in direction of motion of a body takes place only if some external force acts on the body. This external force which is responsible to move a body in a circle with uniform speed is called Centripetal Force.
It follows that a body in uniform circular motion is in a continuous accelerated motion and acceleration is directed towards the centre of circle along the radius of circular path. It is called Centripetal acceleration and it is given by

where ‘r’ is radius of circular path and ‘v’ is the velocity of moving body.Therefore centripetal force required to move a body of mass M with uniform speed ‘v’ along a circular path is given by :
F = Mass x Acceleration
Or
![]()
Since ,
![]()
![]()
Example of Centripetal force
When a car takes a turn on a curved road, it requires centripetal force. This force is provided by the frictional force between the tyres and the road. If the tyres are weared or the road is wet, the frictional force is reduced. If it is too small to provide the necessary centripetal force, the car starts slipping instead of taking steady turn.
References
- Nootan Physics by kumar – Mittal (Part I)
- Modern’s abc of Physics (Vol. I)
